All statistics and graphs for Type 1 Gage Study

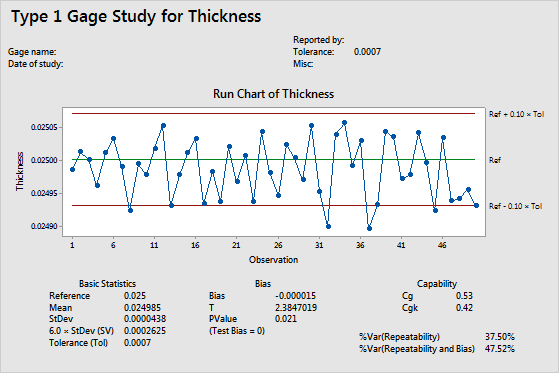
The run chart shows how the measurements vary with respect to the reference value and the tolerance range. The observations are plotted in measurement sequence. Use a run chart to look for evidence of bias or other measurement system variation in your process.

On the run chart, the plotted points represent the individual measurement values of the standard part in the order that they were measured. The green horizontal reference line (Ref), represents the reference value of the standard that you specify. The red horizontal lines are calculated from the reference value and 10% of the tolerance. The area between the red lines represents 20% of the tolerance range (± 10% of the tolerance range). When all the plotted points are within ± 10% of the tolerance range, the measurement system is capable.

The reference value is the known and correct measurement for the standard part. It serves as a master value for comparison during measurement system analysis. For example, you have a reference part with a known weight of 0.025g that you use to calibrate your scales.

Ideally, the reference value should be close to the center of the tolerance zone for the characteristic that you measure.

Reference values can be determined in many ways, depending on industry standards and company and customer expectations. Usually a reference value is determined by taking an average of repeated measurements from more accurate measuring equipment, or by using a lab-certified standard.

The mean is the average of all the measurements of the standard, which is the sum of all the measurements divided by the number of measurements.

StDev is the standard deviation of all the measurements of the standard.

The standard deviation is the most common measure of dispersion, or how spread out the data are from the mean. A larger sample standard deviation indicates that your data are spread more widely around the mean.

The study variation is 6 times the standard deviation.

By default, the study variation for each component is six times its standard deviation, but you can change the multiplier. AIAG recommends the use of 6 in gage R&R studies because 6 is the number of standard deviations needed to capture 99.73% of the variation from a source. For instance, specify 5.15 to capture 99% of the variation.

The tolerance is the tolerance range of the measured component. It is the difference between the upper and lower specification limits.

The bias is a measure of a measurement system's accuracy. Bias is calculated as the difference between the known standard value of a reference part and the observed average measurement.

T is the t-statistic for the alternative hypothesis that bias ≠ 0.

The t-test compares this observed t-statistic to a critical value on the t-distribution with (n-1) degrees of freedom to determine whether the bias in the measurement system is statistically significant.

The p-value is associated with the t-statistic. It is the probability of obtaining a t-statistic as large or larger than the calculated one, assuming that the bias is zero. As the t-statistic increases, the p-value decreases. A small p-value implies that the assumption that the bias = 0 is probably not true.

C_g is a capability index that compares the tolerance range with the measurement variation from the gage and the operator.

C_gK is a capability index that compares the tolerance range with the total of the bias and the measurement variation from the gage and the operator.

The resolution is the specified gage resolution.

A guideline for the resolution is that it should not be greater than 5% of the tolerance. So, if you specify both the resolution and the tolerance, Minitab calculates whether the resolution is less than, greater than, or equal to 5% of the tolerance.

%Var for repeatability compares the gage repeatability with the tolerance.

%Var for repeatability and bias compares the gage repeatability and bias with the tolerance.