What is a type 1 gage study?
A type 1 gage study assesses only the variation that comes from the gage. Specifically, this study assesses the effects of bias and repeatability on measurements from one operator and one reference part.
When should I use a type 1 gage study?
Use a type 1 gage study at the start of your measurement systems analysis, to focus only on the gage, not on any other sources of variation. In fact, many organizations require a type 1 gage study as the first step in a complete measurement system analysis.
After you certify the gage itself with a type 1 gage study, use Minitab's other gage study tools to broaden your MSA and consider other sources of measurement variation.
Minitab measures repeatability with the Cg metric
Repeatability is the gage's ability to make consistent measurements of the same part. Some measurement variation will be present even in a capable gage, but if the variation is too large in relation to the part's tolerance, the gage will be too variable for its purpose. For example, if the tolerance for a cylinder's diameter is 5mm, but repeated measurements of a reference cylinder also span 5mm, you cannot trust the gage to determine whether a cylinder falls within the tolerance range. The variation of a gage's measurements should be small compared to the tolerance.
To assess a gage's repeatability, Minitab calculates the Cg metric to compare the study variation (the spread of the gage's measurements) with a percentage of the tolerance. Values of Cg greater than 1.33 indicate the spread of the gage's measurements is adequately narrow in relation to your tolerance range.
For example, with the default values of K and L, a Cg metric of 2 indicates that 20% of your tolerance range will cover the entire spread of measurements twice over. This Cg value indicates the gage's effectiveness within this tolerance range.
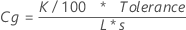
| Term | Description |
|---|---|
| K | percentage of the tolerance (20 is the default) |
| s | standard deviation of measurements |
| L | number of standard deviations that represent the entire process spread (6 is the default) |
Minitab measures bias with the Cgk metric
In addition to repeatability, Minitab also assesses the gage's bias, which is the difference between the gage's average measurement and the official reference value – the "true" value that your gage targets. Minitab analyzes bias with a t-test of the null hypothesis that no bias exists. Essentially, this test is a 1-sample t-test to determine whether the mean measurement differs significantly from the reference value. If a statistically significant bias exists, you conclude your gage is consistently measuring higher or lower than the correct value.
Minitab also calculates the capability metric Cgk to assess repeatability and bias together. Cgk compares the Study Variation to the tolerance, but it also considers whether the measurements are "on target". Cgk decreases as the difference between the gage's mean measurement and the reference value increases. A Cgk value of 1.33 is a common benchmark value to denote a capable gage – one that is both precise (good repeatability) and accurate (low bias).

| Term | Description |
|---|---|
| K | percentage of the tolerance (20 is the default) |
| Xbarg | the mean of all measurements |
| Xm | the reference value |
| s | standard deviation of measurements |
| L | the number of standard deviations you want to represent half the process spread (3 is common) |
