In This Topic
Step 1: Check for possible carryover or period effects
Important
If either the carryover effect or the period effect is statistically significant, you should not evaluate equivalence because your results may be biased by these effects.
Use the p-value for each effect to determine whether it is statistically significant. If the p-value for an effect is below the alpha level (typically, 0.05), then the effect is significant.
Effects
| Effect | SE | DF | T-Value | P-Value | 95% CI for Equivalence | |
|---|---|---|---|---|---|---|
| Carryover | 0.45181 | 0.64988 | 15 | 0.69521 | 0.498 | (-0.93339, 1.8370) |
| Treatment | -0.32104 | 0.060641 | 15 | -5.2941 | 0.000 | (-0.45030, -0.19179) |
| Period | -0.097708 | 0.060641 | 15 | -1.6112 | 0.128 | (-0.22696, 0.031546) |
Key Results: P-values for Effects
In these results, the p-value for the carryover effect (0.498) and the p-value for the period effect (0.128) are both greater than 0.05. Therefore, these effects are not significant at the 0.05 level.
Step 2: Determine whether the test mean and the reference mean are equivalent
Compare the confidence interval with the equivalence limits. If the confidence interval is completely within the equivalence limits, you can claim that the mean of the test population is equivalent to the mean of the reference population. If part of the confidence interval is outside the equivalence limits, you cannot claim equivalence.
Difference: Mean(Generic) - Mean(Brand)
| Difference | SE | 95% CI for Equivalence | Equivalence Interval |
|---|---|---|---|
| -0.32104 | 0.060641 | (-0.427349, 0) | (-0.425035, 0.425035) |
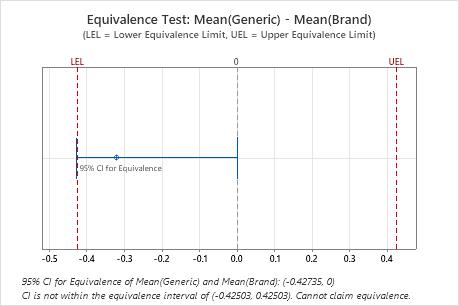
Key Results: 95% CI, Equivalence interval
In these results, the 95% confidence interval is not completely within the equivalence interval defined by the lower equivalence limit (LEL) and the upper equivalence limit (UEL). The lower bound of the confidence interval extends beyond the lower equivalence limit. Therefore, you cannot conclude that the test mean is equivalent to the reference mean.
