In This Topic
Dunnett method for comparisons with a control in a mixed effects model
For multiple comparisons with a control, Minitab also calculates one-sided intervals.
- Upper bound
- Lower bound
For more information on how to calculate the fitted means and the standard error of the difference, go to Methods and formulas for fitted means in Fit Mixed Effects Model.
Note
Calculations for the test statistic, adjusted p-value, individual confidence level, and grouping information table match the calculations for general linear models. For more information, go to the Methods and Formulas for comparisons for general linear models.
Critical value
- Dunnett, C. W. (January 01, 1955). A multiple comparison procedure for comparing several treatments with a control. Journal of the American Statistical Association, 50, 1096-1121.
- J.C. Hsu (1996). Multiple Comparisons: Theory and methods. Chapman & Hall.
| Term | Description |
|---|---|
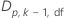 | the upper  percentile of the distribution that Dunnett proposes with  comparisons and df degrees of freedom |
| α | the simultaneous probability of making a Type I error |
| k | the number of levels in the fixed effect term or the random term |
| df | the degrees of freedom |
The degrees of freedom depend on whether the comparison is for a fixed effect term or a random term.
Degrees of freedom (df)
For a fixed effect term, the degrees of freedom (df) are the same as the degrees of freedom for testing the corresponding fixed effect term. For a random term, the degrees of freedom use Satterthwaites approximation method.
For more information on the calculation of the degrees of freedom, go to Methods and formulas for tests of fixed effects in Fit Mixed Effects Model.
Fisher method for comparisons with a control in a mixed effects model
For multiple comparisons with a control, Minitab also calculates one-sided intervals.
- Upper bound
- Lower bound
For more information on how to calculate the fitted means and the standard error of the difference, go to Methods and formulas for fitted means in Fit Mixed Effects Model.
Note
Calculations for the test statistic, adjusted p-value, individual confidence level, and grouping information table match the calculations for general linear models. For more information, go to the Methods and Formulas for comparisons for general linear models.
Critical value
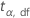
| Term | Description |
|---|---|
 | the  quantile from the student's t distribution with df degrees of freedom |
| α | the individual probability of making a Type I error |
| df | the degrees of freedom |
The degrees of freedom depend on whether the comparison is for a fixed effect term or a random term.
Degrees of freedom (df)
For a fixed effect term, the degrees of freedom (df) are the same as the degrees of freedom for testing the corresponding fixed effect term. For a random term, the degrees of freedom use Satterthwaites approximation method.
For more information on the calculation of the degrees of freedom, go to Methods and formulas for tests of fixed effects in Fit Mixed Effects Model.
Bonferroni method for comparisons with a control in a mixed effects model
For multiple comparisons with a control, Minitab also calculates one-sided intervals.
- Upper bound
- Lower bound
For more information on how to calculate the fitted means and the standard error of the difference, go to Methods and formulas for fitted means in Fit Mixed Effects Model.
Note
Calculations for the test statistic, adjusted p-value, individual confidence level, and grouping information table match the calculations for general linear models. For more information, go to the Methods and Formulas for comparisons for general linear models.
Critical value
| Term | Description |
|---|---|
 | the upper  percentile of the student's t distribution with df degrees of freedom |
| α | the simultaneous probability of making a Type I error |
| c |  |
| k | the number of levels in the fixed effect term or the random term |
| df | the degrees of freedom |
The degrees of freedom depend on whether the comparison is for a fixed effect term or a random term.
Degrees of freedom (df)
For a fixed effect term, the degrees of freedom (df) are the same as the degrees of freedom for testing the corresponding fixed effect term. For a random term, the degrees of freedom use Satterthwaites approximation method.
For more information on the calculation of the degrees of freedom, go to Methods and formulas for tests of fixed effects in Fit Mixed Effects Model.
Sidak method for comparisons with a control in a mixed effects model
For multiple comparisons with a control, Minitab also calculates one-sided intervals.
- Upper bound
- Lower bound
For more information on how to calculate the fitted means and the standard error of the difference, go to Methods and formulas for fitted means in Fit Mixed Effects Model.
Note
Calculations for the test statistic, adjusted p-value, individual confidence level, and grouping information table match the calculations for general linear models. For more information, go to the Methods and Formulas for comparisons for general linear models.
Critical value
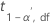
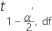
| Term | Description |
|---|---|
 | the upper  percentile of the student's t distribution with df degrees of freedom |
 |  |
| α | the simultaneous probability of making a Type I error |
| c |  |
| k | the number of levels in the fixed effect term or the random term |
| df | the degrees of freedom |
The degrees of freedom depend on whether the comparison is for a fixed effect term or a random term.
Degrees of freedom (df)
For a fixed effect term, the degrees of freedom (df) are the same as the degrees of freedom for testing the corresponding fixed effect term. For a random term, the degrees of freedom use Satterthwaites approximation method.
For more information on the calculation of the degrees of freedom, go to Methods and formulas for tests of fixed effects in Fit Mixed Effects Model.
