Uses of the exponential distribution to model reliability data
The exponential distribution is a simple distribution with only one parameter and is commonly used to model reliability data. The exponential distribution is actually a special case of the Weibull distribution with ß = 1.
- The exponential distribution is frequently used to model electronic components that usually do not wear out until long after the expected life of the product in which they are installed. Examples include components of high-quality integrated circuits, such as diodes, transistors, resistors, and capacitors.
- The exponential distribution is also considered an excellent model for the long, "flat"(relatively constant) period of low failure risk that characterizes the middle portion of the Bathtub Curve. This phase corresponds with the useful life of the product and is known as the "intrinsic failure" portion of the curve.
- However, the exponential distribution should not be used to model mechanical or electric components that are expected to show fatigue, corrosion, or wear before the expected life of the product is complete, such as ball bearings, or certain lasers or filaments.
An important property of the exponential distribution is that it is memoryless. The memoryless property indicates that the remaining life of a component is independent of its current age. For example, a system that is subjected to wear and tear and thus becomes more likely to fail later in its life is not memoryless. Therefore, this distribution should be used when the failure rate is constant during the entire life of the product. The number of failures per unit in time is usually expressed as percent of failure per unit time, such as percent of failure per thousand hours.
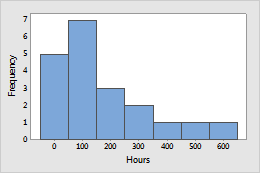
Example 1: Transistors
An electronic component is known to have a constant failure rate during the expected life of a product. Engineers record the time to failure of the component under normal operating conditions.
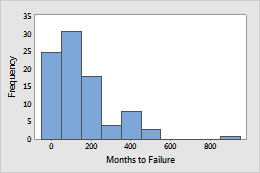
Example 2: Filaments
A light bulb company manufactures incandescent filaments that are not expected to wear out during an extended period of normal use. They want to guarantee it for 10 years of operation. Engineers stress the bulbs to simulate long-term use and record the months until failure for each bulb.
Probability density function and hazard function for the exponential distribution
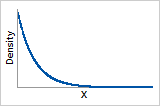
Probability density function
The probability density function shows that the failure data are skewed to the right

Hazard function
The hazard function shows that the risk of failure is constant
