Kaplan-Meier estimation

The Kaplan-Meier estimator, also known as the product limit estimator, can be used to calculate survival probabilities for nonparametric data sets with multiple failures and suspensions. The equation of the estimator is given by:

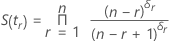
with S(t₀) = 1 and t₀ = 0.

Empirical hazard function

The hazard function describes the rate of failure for an interval. The hazard function is 0 before the first censored observation. The hazard function changes only at uncensored observations. Minitab does not plot the hazard function after the last uncensored data point.

When there are ties, Minitab uses the largest rank in the tie to estimate the hazard function. See Nelson¹ for more details.

Mean time to failure

For uncensored data, the mean time to failure is the same as the average failure time. The general formula to use with censored or uncensored data follows:

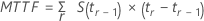
Also, when the largest observation is censored, Minitab treats the time of the largest uncensored observation as a time limit for the calculation. See Lee² for more details.

Standard error of MTTF

The standard error of the mean time to failure is the square root of the variance. When all observations are uncensored, Minitab calculates an unbiased estimate:

For the cases where some data are censored, the unbiased estimate of the variance is the following formula:

Because of the shape of the empirical hazard function, the areas under the survival curve, A_r, are rectangles with heights equal to the survival function and lengths equal to the intervals between uncensored observations.

Notation

Term	Description
tr	time of the data point with rank r
r	rank of the data point, where the earliest failure has the lowest rank
n	total number of units
δr	0 if the jth observation is censored or 1 if the jth observation is uncensored
c	number of data points until the next uncensored observation
S(tr)	empirical survival function at time tr
	average failure stress
Ar	area under the curve of the survival plot to the right of tr
m	total number of uncensored observations

References

1. W. Nelson (1982). Applied Life Data Analysis. John Wiley & Sons, Inc. 133.

2. Elisa T. Lee (1992). Statistical Methods for Survival Data Analysis, Second Edition. John Wiley & Sons, Inc. 73-76.