The actuarial model is an alternative nonparametric analysis that displays information for groupings of failure times. The Kaplan-Meier method assumes that the suspensions in an interval occur at the end of that interval, after the failures have occurred. Minitab's actuarial model assumes that the suspensions occur in the middle of the interval, which has the effect of reducing the number of available units in the interval. The estimate of the survival function using the actuarial method is as follows:
 for i = 0
for i = 0
 for i > 0
for i > 0
Empirical hazard function
The hazard function describes the rate of failure for an interval. With actuarial estimation, you assume that the calculation is for the midpoint of the interval. On the hazard plot, the function is drawn from midpoint to midpoint. For more details, see the references following the Notation section.
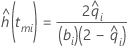
Notation
| Term | Description |
|---|---|
| ni | the number of units entering an interval |
| di | the number failing in the interval |
| n'i |  |
 | the number censored in an interval |
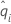 | the conditional probability of an event, which equals di/n'i |
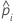 |  |
| tmi | time at the midpoint of the actuarial interval |
| bi | the length of the actuarial interval |
References
Collett, D. (1994) Modelling Survival Data in Medical Research, Chapman and Hall.
Lee, Elisa T. (1992) Statistical Methods for Survival Data Analysis, 2nd Edition, John Wiley & Sons.
