All product performance reports for Product Report

In This Topic

Report 7: Product Performance
Report 8A: Product Benchmarks (DPMO versus Z.Bench)
Report 8B: Product Benchmarks (Z.Shift versus Z.Bench)
Report 8C: Product Benchmarks (Capability, Complexity, Control)

Report 7: Product Performance

The Product Performance report calculates the rolled up performance measures when the lower level elements are combined into a higher level unit.

Six Sigma Product Report

Rollup Statistics Opps Obs Obs per Adj Adj Total Component Defs Units Unit Cmplx Adj Defs Units Opps DPU 1 77 184 56 1 32.641 78 4368 0.418478 2 3 907 95 6 1.548 468 44460 0.003308 3 59 750 59 5 30.680 390 23010 0.078667 4 28 567 79 4 15.407 312 24648 0.049383 5 73 829 64 5 34.343 390 24960 0.088058 6 28 132 30 1 16.545 78 2340 0.212121 7 1 547 76 3 0.428 234 17784 0.001828 8 5 726 30 5 2.686 390 11700 0.006887 9 2 78 28 1 2.000 78 2184 0.025641 10 89 655 55 4 42.394 312 17160 0.135878 11 74 715 98 5 40.364 390 38220 0.103497 12 3 453 36 3 1.550 234 8424 0.006623 13 99 233 10 1 33.142 78 780 0.424893 14 49 726 80 5 26.322 390 31200 0.067493 15 78 832 81 5 36.563 390 31590 0.093750 16 50 783 1 5 24.904 390 390 0.063857 17 88 807 10 5 42.528 390 3900 0.109046 18 61 123 40 1 38.683 78 3120 0.495935 19 4 906 57 6 2.066 468 26676 0.004415 20 21 696 48 5 11.767 390 18720 0.030172 Total 436.561 78 335634

Component DPMO Z.Shift Z.ST YTP YRT 1 7472.8 1.500 3.934 0.657014 0.657014 2 34.8 1.500 5.478 0.996698 0.980350 3 1333.3 1.500 4.504 0.924299 0.674627 4 625.1 1.500 4.727 0.951802 0.820704 5 1375.9 1.500 4.494 0.915652 0.643655 6 7070.7 1.500 3.954 0.808257 0.808257 7 24.1 1.500 5.565 0.998173 0.994530 8 229.6 1.500 5.004 0.993136 0.966147 9 915.8 1.500 4.616 0.974673 0.974673 10 2470.5 1.500 4.311 0.872802 0.580315 11 1056.1 1.500 4.574 0.901630 0.595856 12 184.0 1.500 5.062 0.993399 0.980327 13 42489.3 1.500 3.223 0.647793 0.647793 14 843.7 1.500 4.640 0.934708 0.713475 15 1157.4 1.500 4.547 0.910461 0.625614 16 63857.0 1.500 3.023 0.936143 0.718970 17 10904.6 1.500 3.794 0.896152 0.577976 18 12398.4 1.500 3.745 0.607116 0.607116 19 77.5 1.500 5.283 0.995595 0.973857 20 628.6 1.500 4.726 0.970269 0.859926 Total 1300.7 1.500 4.511 0.003598

Component

Optional column to provide names for components. If you don't specify names, Minitab assign numbers as IDs.

Obs Defs

Number of defects observed.

Obs Units

Number of units of each component observed.

Opps per Unit

Number of opportunities (for defect) per unit.

For more information, go to What is opportunities per unit?.

Cmplx

Complexity counts for each component. You can adjust the observed units and observed defects by setting proportions for each component. For example, to make a larger assembly, you need 1 unit of component 1, 6 units of component 2, 5 units of component 3, and so on.

The complexity column is not required, but using complexity values reduces the effects of disproportionate sampling. If there are no proportions, enter a column of all 1s.

For more information, go to What is complexity?.

Adj Defs

Observed defects are adjusted (or weighted) based on the complexity information. When no complexity units are given, adjusted defects are the same as observed defects.

Adj Units

Observed units are adjusted (or weighted) based on the complexity information. When no complexity units are given, adjusted units are the same as observed units. All components that have the same complexity have the same adjusted units. In the example, components 7 and 12 both have complexity of 3 and adjusted units of 234.

Adj Total Opps

This column is calculated by multiplying Adj Units and Opps per Unit. If the unit counts were not adjusted, the total opportunities would be skewed in favor of components with larger numbers of observed units, which would affect the calculations of performance statistics. Again, using complexity values reduces the effects of disproportionate sampling.

DPU

Defects per unit, calculated by dividing the number of defects by the number of units.

DPMO

Defects per million opportunities, calculated by dividing Adj Units by Adj Tot Opps, then multiplying by 1 million.

If the unit counts were not adjusted, the total opportunities would be skewed in favor of components with larger numbers of observed units.

Z.Shift

Values to represent the assumed long-term sigma shift. If one is not specified, Minitab uses the default values of 1.5.

For more information, go to Z.bench as an estimate of sigma capability.

Z.ST

Z scores calculated from DPMO and Z.Shift.

YTP

Throughput yield for each component. This is the probability that none of the opportunities in the component result in a defect.

For more information, go to What are throughput yield (YTP) and rolled throughput yield (YRT)?.

YRT

Rolled throughput yield for each component. The probability of having one good unit of component 2 is the YTP, 0.996698. To make a larger assembly, you need 6 units of component 2. The probability of having 6 good units of component 2 is the YRT, (0.996698)⁶ = 0.980350.

For more information, go to What are throughput yield (YTP) and rolled throughput yield (YRT)?.

Report 8A: Product Benchmarks (DPMO versus Z.Bench)

The Product Benchmarks (DPMO versus Z.Bench) report displays a graphical view of the benchmark statistics for the collection of components that are in the product report.

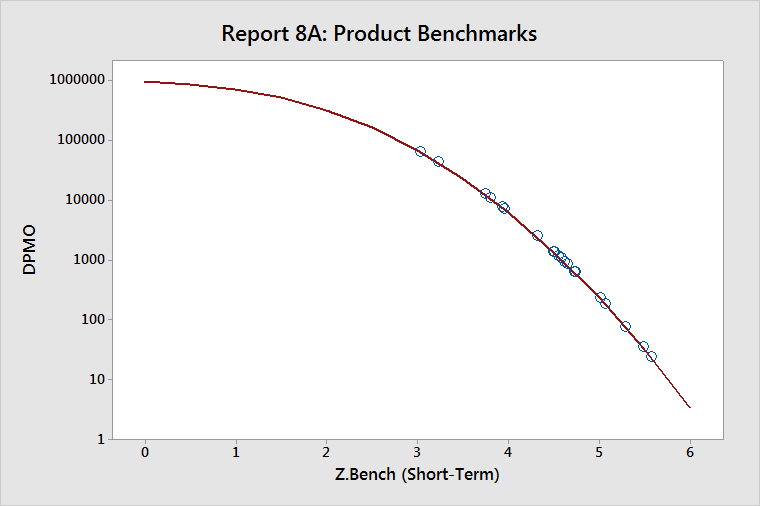
DPMO is a measure of long-term performance. Z.Bench ST is a measure of short-term performance.

The locations of the clusters of points represent where the capabilities of your processes tend to be concentrated. In the example above, there is a cluster just below 4 on the Z.ST scale, and another near 4.5. Thus, many of the processes used here run from just under 4 to just over 4 sigma. This is quite typical.

Report 8B: Product Benchmarks (Z.Shift versus Z.Bench)

The Product Benchmarks (Z.Shift versus Z.Bench) report displays another view of the benchmark statistics for the collection of components that are in the product report.

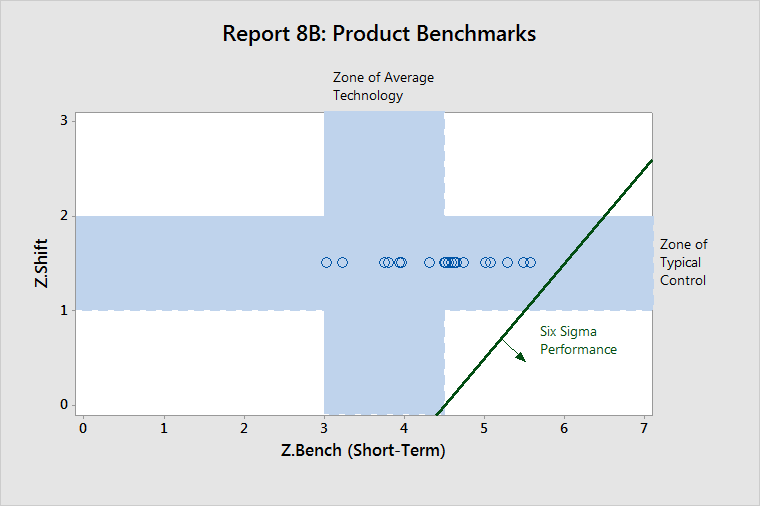
This graph compares the controllability of each component (Z.Shift) and the capability of each component (Z.ST). Typically, Z.Shift values fall within the horizontal band (Zone of Typical Control) and Z.ST values fall within the vertical band (Zone of Average Technology).

Six Sigma Performance is achieved at high levels of Z.Bench and low levels of Z.Shift.

Z.Shift

Low Z.Shift values correspond to characteristics that are very well controlled.
High Z.Shift values correspond to characteristics that are poorly controlled.

Z.Bench ST

High Z.Bench values correspond to characteristics that represent technological superiority.
Low Z.Bench values correspond to characteristics that represent technological inferiority.

In the example above, all components had a Z.shift of 1.5 sigma, which is the default when actual Z.Shift values are unknown. About half of the components have Z.Bench values in the Zone of Average Technology. The other half of the components have values to the right, which indicates better than average capability.

Report 8C: Product Benchmarks (Capability, Complexity, Control)

Without complexity information

When you do not include complexity information, the Product Benchmarks report includes the following graphs:

YTP: A reverse Pareto diagram of throughput yields (YTP)
Opps per Unit: Opportunities per unit for the components, ordered by YTP values
Z.ST: Z.ST values for the components, ordered by YTP values
Z.Shift: Z.shift values for the components, ordered by YTP values

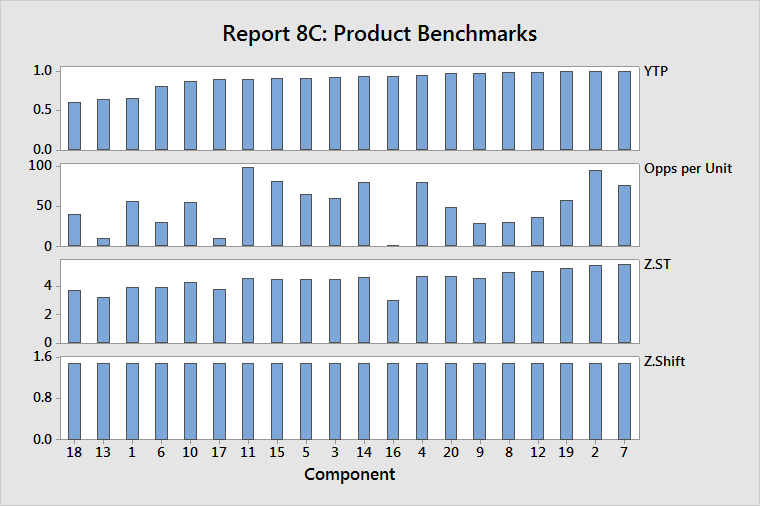
In the YTP graph, identify the components that have the worst quality, then look at the lower charts to determine whether the problem is a result of high complexity (opportunity count), low capability (Z.ST), or poor control (Z.Shift).

In the example above, component 18 has the worst quality, moderate opportunity count, and below average capability. Improving capability will have the largest impact on improving quality.

With complexity information

When you include complexity information, the Product Benchmarks report includes the following graphs:

YRT: A reverse Pareto diagram of rolled throughput yields (YRT)
Total Opps per Unit: Opportunities per unit for the components, ordered by YRT values
Z.ST: Z.ST values for the components, ordered by YRT values
Z.Shift: Z.shift values for the components, ordered by YRT values

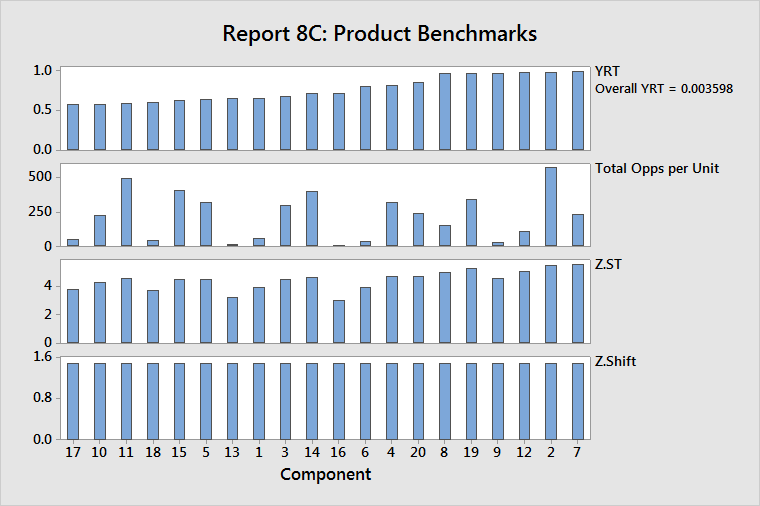
Remember, the overall YRT represents the probability that a single unit of the entire collection of components can be produced without any defects. The components that have the lowest component-level YRT values contribute the most to the overall YRT. Thus, improving those components is critical for improving overall YRT.

In the example above, component 17 has the lowest YRT, a low opportunity count, and low capability. Raising the average Z.ST for component 17 will have the largest effect on improving its quality and, thereby, improving the overall product quality.

Component 11 (the third worst component) has a high opportunity count and good capability. Reducing the opportunity count will have the largest effect on improving the quality of component 11, because the capability is already quite good.