Plotted points
The plotted points on the G chart are the number of opportunities between events or the days between events.
Interpretation
If the process is in control, the points vary randomly around the center line, and the process exhibits only common-cause variation. Investigate points that fail any of the tests for special causes or that exhibit nonrandom patterns for possible special-cause variation.
Center line
The center line on the G chart is the 50th percentile of the distribution.
Interpretation
Use the center line to observe how the process performs compared to the average. If the process is in control, then the points vary randomly around the center line.
CAUTION
Do not confuse the center line with the target value for your process. The target is your desired outcome. The center line is the actual outcome.
Control limits
Control limits are the horizontal lines that are above and below the center line. On a G chart, the lower control limits is always set to 0. The upper control limit indicates whether a process is out of control, and it is based on the inverse CDF of the geometric distribution.
Tests for special causes
The tests for special causes assess whether the plotted points are randomly distributed within the control limits.
Interpretation
Use the tests for special causes to determine which observations you may need to investigate and to identify specific patterns and trends in your data. Each of the tests for special causes detects a specific pattern or trend in your data, which reveals a different aspect of process instability. For example, Test 1 detects a single out-of-control point. Test 2 detects a possible shift in the process.
Four tests are available with this control chart.
- Test 1: One point more than 3σ from center line
- Test 1 identifies subgroups that are unusual compared to other subgroups. Test 1 is universally recognized as necessary for detecting out-of-control situations. If small shifts in the process are of interest, you can use Test 2 to supplement Test 1 in order to create a control chart that has greater sensitivity.

- Benneyan Test: Successive point equal to 0
- The Benneyan test counts the number of consecutive plotted points that are equal to 0. When a point on a G chart fails the Benneyan test, the point is denoted with B. The number of points that are required to signal the Benneyan test is a function of the desired false alarm rate and the event probability. The false alarm rate is based on the probability that is associated with the test 1 argument, which is 3 by default.
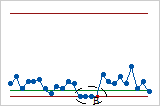
- Test 2: Nine points in a row on the same side of the center line
- Test 2 identifies shifts in the process variation. If small shifts in the process are of interest, you can use Test 2 to supplement Test 1 in order to create a control chart that has greater sensitivity.

- Test 3: Six points in a row, all increasing or all decreasing
- Test 3 detects trends. This test looks for a long series of consecutive points that consistently increase in value or decrease in value.
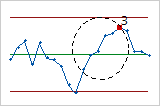
- Test 4: Fourteen points in a row, alternating up and down
- Test 4 detects systematic variation. You want the pattern of variation in a process to be random, but a point that fails Test 4 might indicate that the pattern of variation is predictable.

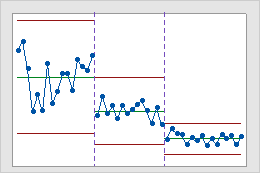
Stages
Use stages to create a historical control chart that shows how a process changes over specific periods of time. By default, Minitab recalculates the center line and control limits for each stage. For more information, go to Add stages to show how a process changed.
Interpretation
This historical control chart shows three stages of a process, which represent before, during, and after the implementation of a new procedure.